
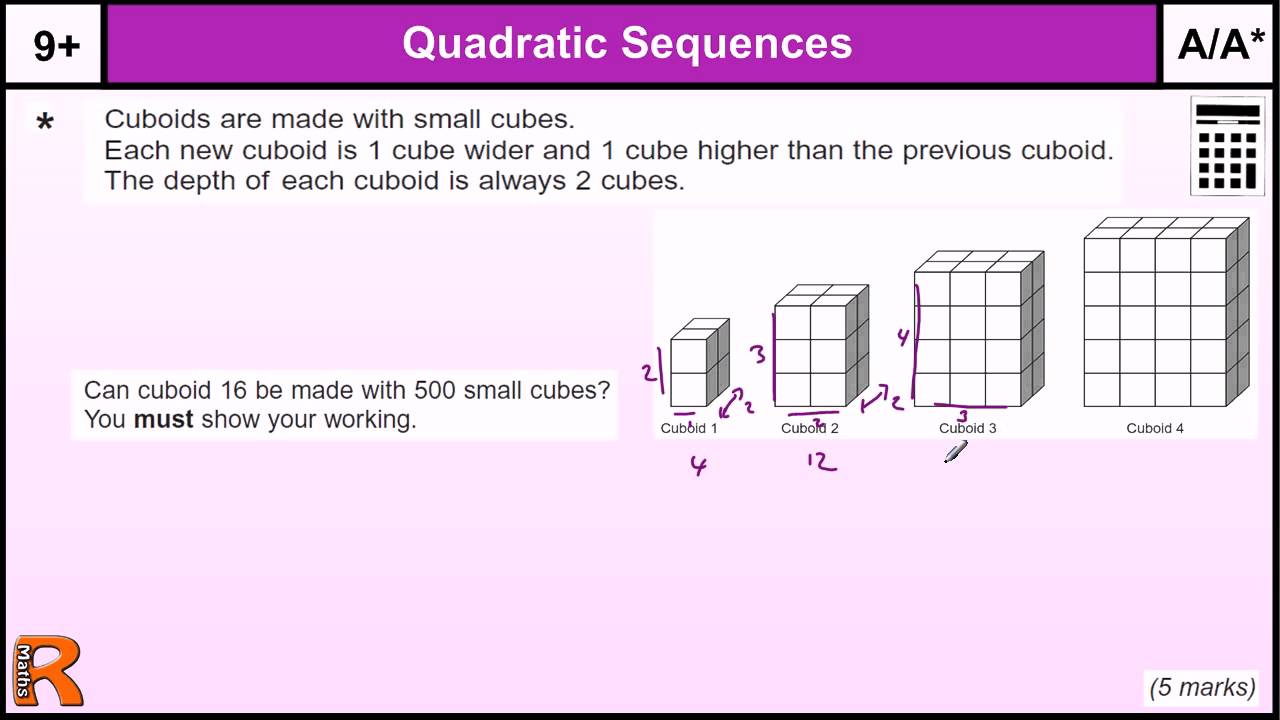
n represents the term (position) numbers and therefore it can only be positive integers starting from 1 and should also not include 0, n=1, 2, 3, 4, 5, …. Quadratic sequences sequences wall displayĮnter your email address to follow this blog and receive notifications of new posts by email.A common error is to forget to half the second difference before using it as the coefficient of n^ = −1, −4, −9, −16, … has a second difference of −2 but is incorrectly written as 2.
Quadratic sequence pdf#
Along with Stepwise Solutions, Timing, PDF download to boost your the GCSE Maths. The Sequence Calculator finds the equation of the sequence and also allows you to view the next terms in the sequence. Must Practice GCSE (9-1) Maths Quadratic Sequences Past Paper Questions.
Quadratic sequence series#
On quadratic sequences specifically, a nice worksheet here from back to Don Steward with this which is actually just a series of terms stacked on top of each other. Step 1: Enter the terms of the sequence below. Get your students to create their own once they have got the idea.
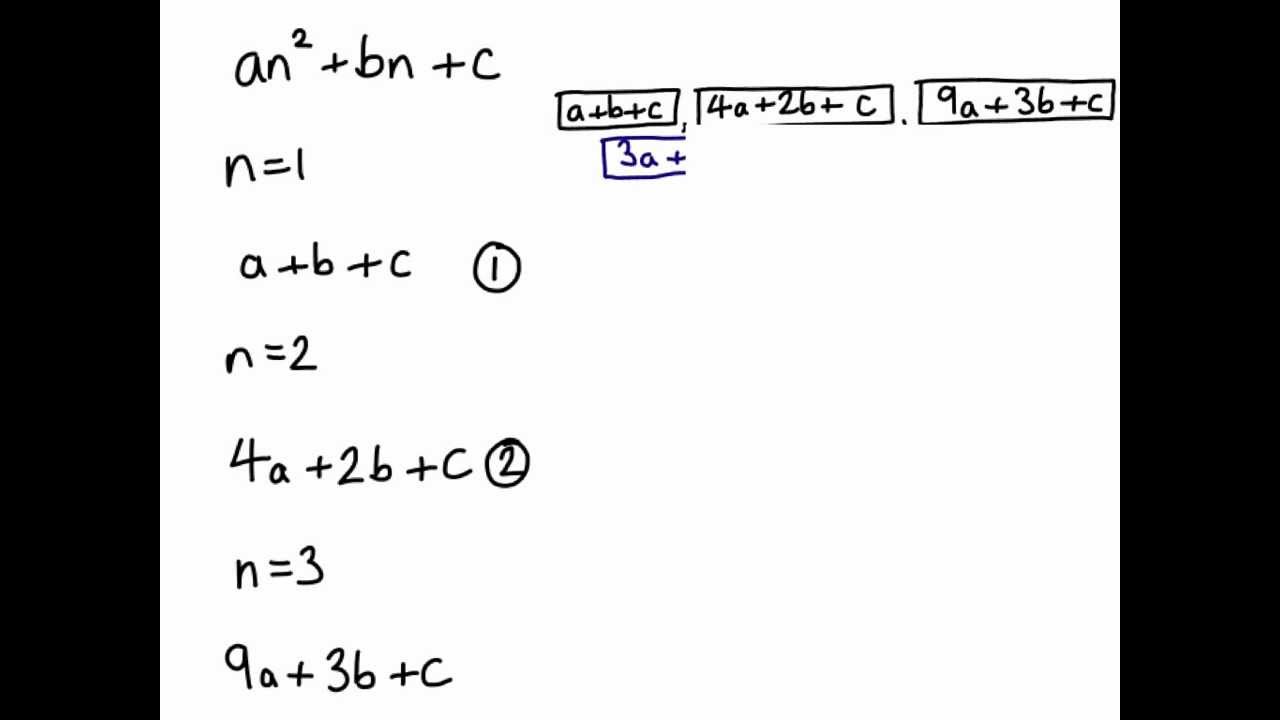
There are also over a hundred sequences at a website entirely dedicated to, well, visual patterns. since the sequence is quadratic, you only need 3 terms. We say that the second difference is constant.

Consequently, the 'difference between the differences between the sequence's terms is always the same'. It is written in the form: ax2 + bx + c 0 where x is the variable, and a, b, and c are constants, a 0. Quadratic sequences of numbers are characterized by the fact that the difference between terms always changes by the same amount. When the Discriminant ( b24ac) is: positive, there are 2 real solutions. In math, a quadratic equation is a second-order polynomial equation in a single variable. that means the sequence is quadratic/power of 2. Quadratic Equation in Standard Form: ax 2 + bx + c 0. This is nice: one way we can see the sequence of square numbers, the other way we can see how multiplying one dimension by the other leading to some brackets which can be multiplied out. however, you might notice that the differences of the differences between the numbers are equal (5-32, 7-52). We can then extend this on to quadratic sequences. However, there are a couple of ways you could look at this which combine sequences which are arguably simpler. There are also infinitely many quadratic polynomials that obtain at least one prime value, e.g. For example, the following patterns produce a linear sequence. There are infinitely many linear polynomials f(n) an + b f ( n) a n + b such that f(n) f ( n) yields prime values at infinitely many integer arguments - I assume this is what 'producing primes' means. It got me thinking about using shapes to represent sequences and in particular using different colours to represent different sequences laid on top of each other. After browsing the ever excellent Don Steward’s Median site for sequences, I found this on Linear and Quadratic growths.


 0 kommentar(er)
0 kommentar(er)
